(Source: Herbert Goldstein, Classical Mechanics - Chapter 01)
Kinetic Energy:
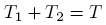
Where T1 equals the kinetic energy of the center of mass, and T2 is the kinetic energy about the center of mass. Keep these two parts seperate!
Solve for T1 first, its the easiest:
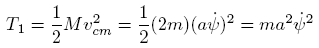
Solve for T2, realizing that the rigid rod is not restricted to just the X-Y plane. Don't forget the Z-axis!
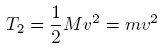
Solve for v2 about the center of mass. The angle
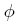 will be the angle in the x-y plane, while the angle
will be the angle in the x-y plane, while the angle  will be the angle from the z-axis.
will be the angle from the z-axis.If
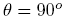 and
and 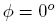 then x = l/2 so:
then x = l/2 so: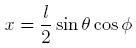
If
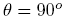 and
and 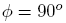 then y = l/2 so:
then y = l/2 so:
If
 = 0, then z = l/2 so:
= 0, then z = l/2 so: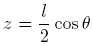
Find v2:
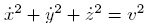
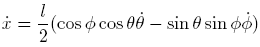
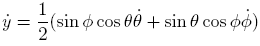
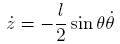
Square each:
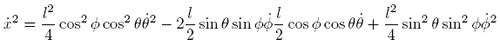
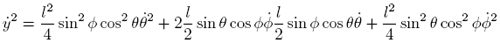
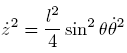
Now add, striking out the middle terms:
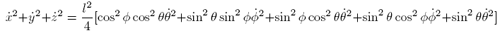
Pull the first and third terms inside the brackets together, and pull the second and fourth terms together as well:
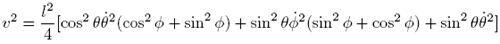

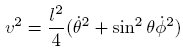
Now that we finally have v2 we can plug this into T2
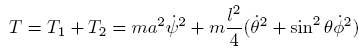
It was important to emphasize that T1 is the kinetic energy of the total mass around the center of the circle while T2 is the kinetic energy of the masses about the center of mass.